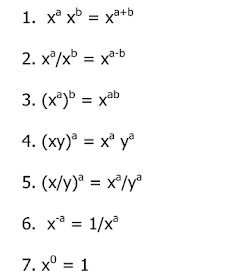I don't usually enjoy teaching properties because they seem so math-y. I like asking my kids to justify what they do, but for many, the properties just seem to be vocabulary that is forced upon them. Necessary evil, I guess? They are great for doing mental math tricks and kids use them without thinking of them, so I suppose there is no harm in giving a name to the stuff they already do.
Raise your hand if your kids mix up associative and commutative properties?
No more.
The Process
1. Give example of property with respect to addition.
ex: Associative: (2 + 3) + 4 = 2 + (3 + 4)
2. Ask students to write another example of their own.
3. Ask for a rule using a, b and c.
4. Ask students to write down the key characteristics of the associative property in Tweet form. (very few words)
Now here's the kicker:
5. Can you guess what the property for multiplication is going to look like?
This worked great for the associative, commutative and identity properties. A great discussion on the inverse property ensued and I ended up telling them that we want a multiplication problem that equals 1.
Done.
None of these properties are worth anything if we don't apply them. Next step is getting them to put words to all that stuff they "just do in my head."
6.
Let's synthesize this a bit more.
7. Now write a similar problem using the multiplicative properties.
I told the class to keep an eye out for times when we will use the inverse and identity properties--which will happen daily once we start solving equations.