Questioning is a huge part of my own classroom but working with my own kids has been a great mirror for me. I missed a few opportunities with my son here. See if you can pick them out.
Pages
▼
Tuesday, June 29, 2010
Monday, June 28, 2010
Adventures in Pedagogy: Motivation
I'm not exactly sure what to make of it, but my children's motivation towards learning can be modeled by y = k/x where y = motivation and x = age. We've got Aidan (5)...
And Nevan (8)...
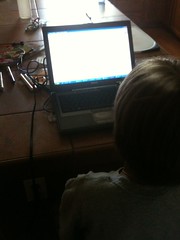
...who was dialed-in to this really simple applet I built for Dawson (11).
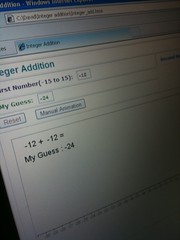
That's not to say Dawson has no motivation, but it's clear that he's been affected by the point- gathering-culture that is school.
Nevan spent a lot of time simply investigating integer addition and it isn't even on his radar right now as far as planned curriculum goes. He played with (+)+(+) and moved on his own to (-)+(-).
During lunch he asked, "Dad, can you teach me to add a positive plus a negative?"
Guess what my answer was. Go ahead, guess.
I built a couple of assessments in ExamView for them and Dawson was all jacked at his instant-feedback-Dad-I-got-em-all-right performance.
Now they're asking for assessments.
We're not paying them, either. With points or anything.
And Nevan (8)...

...who was dialed-in to this really simple applet I built for Dawson (11).

That's not to say Dawson has no motivation, but it's clear that he's been affected by the point- gathering-culture that is school.
Nevan spent a lot of time simply investigating integer addition and it isn't even on his radar right now as far as planned curriculum goes. He played with (+)+(+) and moved on his own to (-)+(-).
During lunch he asked, "Dad, can you teach me to add a positive plus a negative?"
Guess what my answer was. Go ahead, guess.
I built a couple of assessments in ExamView for them and Dawson was all jacked at his instant-feedback-Dad-I-got-em-all-right performance.
Now they're asking for assessments.
We're not paying them, either. With points or anything.
Saturday, June 26, 2010
Problem Solving: Rubric
This one's a follow up to a previous post on which I'd still appreciate some push-back. (So if you haven't read that one, go there first) I'm still convinced that problem-solving/synthesis/application or anything upstream from skill duplication needs to be handled separately from the skill itself. That's not to say that a student can't validate a score on a particular skill(s) based on how they do on a richer assessment, but these types of problems are difficult to reassess for reasons stated in the Taxonomy post. I did some tinkering with a rubric and tried it out with my 7th graders. I liked what I saw.
I'm trying to make my students aware of four things:
Tell me everything you can about the relationship described by the points: (2, 5) (-1, -1) and (5, 11).
...and gave them a page with two copies of this rubric. One for them to fill out when they finished the problem and one for me to fill out after they turned it in.
I'm not completely pleased with this rubric because there will be times when there may not be multiple points of entry to a problem or all four ways to represent a problem may not be applicable. Giving them the rubric was huge. From that day on, student's showing work ceased being a problem. They all realized that everything in class became an opportunity for assessment, I was clear about my expectations and they rose to meet them.
How does this work with SBG?
Each of the four columns will have it's own standard. I may weigh them as I think this is the stuff we're really after. I will also allow a student to call on skills to be validated. For example, if a student can identify finding slope as a skill needed to solve this problem and performs it correctly, the score on the slope standard becomes a 5. I do think that the student needs to identify the skill in order for it to be validated, though.
I know these ideas need some sharpening and I may be missing something, but I'm throwing them out to you anyway. So in all your free time...
I'm trying to make my students aware of four things:
- There's often times more than one way to solve a problem.
- There are usually four ways to represent a problem/solution: verbally, numerically, symbolically and graphically.
- Multiple skills can be put together to create new understanding.
- Strategies can often be generalized into a rule.
Tell me everything you can about the relationship described by the points: (2, 5) (-1, -1) and (5, 11).
...and gave them a page with two copies of this rubric. One for them to fill out when they finished the problem and one for me to fill out after they turned it in.
I'm not completely pleased with this rubric because there will be times when there may not be multiple points of entry to a problem or all four ways to represent a problem may not be applicable. Giving them the rubric was huge. From that day on, student's showing work ceased being a problem. They all realized that everything in class became an opportunity for assessment, I was clear about my expectations and they rose to meet them.
How does this work with SBG?
Each of the four columns will have it's own standard. I may weigh them as I think this is the stuff we're really after. I will also allow a student to call on skills to be validated. For example, if a student can identify finding slope as a skill needed to solve this problem and performs it correctly, the score on the slope standard becomes a 5. I do think that the student needs to identify the skill in order for it to be validated, though.
I know these ideas need some sharpening and I may be missing something, but I'm throwing them out to you anyway. So in all your free time...
Monday, June 21, 2010
Adventures in Pedagogy: Experiments
Homeschooling has been interesting. Suffice it to say, my kids have developed some bad habits very quickly. It's hard to admit that I've let my own children become answer chasers right under my nose. I ask questions and we have a lot of conversations, but in a typical school day, my kids are gone from 8:00am-3:30pm and then have busywork waste-of-time-packet-work, er, homework to do. By the time they were done, there wasn't really any desire to talk about the hoops they were jumping through stuff they were learning.
This morning was fantastic. Nevan, my 8-year-old, was stoked that he had a science experiment to do. He was looking through his text and saw the experiments and just lit up when my wife told him we were actually going to do them.
"Mom, my book at school had all kinds of experiments, but we never got to do them."
Wait, what?
Now, I'm no science teacher, but I couldn't agree more with Shawn's assessment of the situation.
I think the look on this kid's face speaks for itself.
This morning was fantastic. Nevan, my 8-year-old, was stoked that he had a science experiment to do. He was looking through his text and saw the experiments and just lit up when my wife told him we were actually going to do them.
"Mom, my book at school had all kinds of experiments, but we never got to do them."
Wait, what?
Now, I'm no science teacher, but I couldn't agree more with Shawn's assessment of the situation.
I think the look on this kid's face speaks for itself.
Friday, June 18, 2010
My Apologies
...but I can't get this one out of my head:
Not trying to get preachy here, promise. But here's the thing:
We spend all kinds of time discussing how to better educate our students. For what? Aren't we using our content areas as vehicles to produce better citizens? (or at least that's what we say.) It's not about math, English, science, social studies or P. freakin' E. It's about human beings. It's about the dignity of the individual, right? But if we never help students get past the fact the world doesn't revolve around them, what good is it? Why bother?
So if we are trying to help students get past self and become part of a greater good, what is this greater good? Where does faith enter into this equation? Or does it? Should it?
We have problems in our schools. Serious problems. Most of our kids could give a rip about the Pythagorean Theorem, slope-intercept form or whether or not they put their name in the right place on the paper. Our kids are trying to figure out where they fit into this world and they're thinking "if it's not about me, then who's it about, you? Why should I care about you?"
Most of us don't want to discuss our politics let alone our theology. It's too personal. But there's no way it doesn't affect our pedagogy.
Are we really the answer? Or as we become a little more self-aware should that point us to something greater us? If so, where's that in the curriculum? If it's about more than us, yet us is the only thing we can talk about in schools, we're trying to build a fire without oxygen.
"From Wojtyla's perspective, the moral relativism that has resulted from the modern "turn to the subject" is only the product of an anthropological stagnation in the absence of faith. In other words, man turns to himself and "stays" there, failing to see that his own humanity points him beyond himself, failing to see that anthropology points to theology
(Christopher West, Theology of the Body Explained)
Not trying to get preachy here, promise. But here's the thing:
We spend all kinds of time discussing how to better educate our students. For what? Aren't we using our content areas as vehicles to produce better citizens? (or at least that's what we say.) It's not about math, English, science, social studies or P. freakin' E. It's about human beings. It's about the dignity of the individual, right? But if we never help students get past the fact the world doesn't revolve around them, what good is it? Why bother?
So if we are trying to help students get past self and become part of a greater good, what is this greater good? Where does faith enter into this equation? Or does it? Should it?
We have problems in our schools. Serious problems. Most of our kids could give a rip about the Pythagorean Theorem, slope-intercept form or whether or not they put their name in the right place on the paper. Our kids are trying to figure out where they fit into this world and they're thinking "if it's not about me, then who's it about, you? Why should I care about you?"
Most of us don't want to discuss our politics let alone our theology. It's too personal. But there's no way it doesn't affect our pedagogy.
Are we really the answer? Or as we become a little more self-aware should that point us to something greater us? If so, where's that in the curriculum? If it's about more than us, yet us is the only thing we can talk about in schools, we're trying to build a fire without oxygen.
Ground Floor
About three weeks before the end of the school year, I had a meeting with our district's IT director. We had a really good discussion about how our we have committed many resources towards keeping the tech running but nothing in the way of developing a plan for implementation. This conversation quickly turned into the district deciding to free up my afternoons to help with this.
Things I know
Questions I have
I can't imagine the meeting going better. It was great to see someone with 40+ years in education saying that we need to look at what we do and why we do it. Many of the questions I had, she had as well. One thing we knew for sure was that this vision is fluid and we are in the process of creating it. Questions are encouraged. Push back is needed. Transparency is critical.
Lots of rope. Hope I don't hang myself with it.
Things I know
- Technology isn't going to make a bad teacher a good teacher.
- Using tech to do the exact same things we've done without tech isn't productive.
- Teachers don't like change.
- Anything that comes from the district office is treated like a mandate and resisted.
- "How do I use this?" is the wrong question.
- "Why should I use this?" is a better question.
- Professional development in the way of workshops isn't very effective.
- Conversations are.
Questions I have
- Where does assessment/grading fit into this conversation?
- Are we looking to transform what we do in the classroom?
- Why should anyone listen to what I have to say?
- Where do I start?
I'm not really interested in throwing a bunch of tools into the laps of teachers. However, I'm very interested in engaging in conversations with teachers about why we do what we do and questioning whether or not there exists a tool that will help us do things better. I threw a bunch of these questions in the lap of my IT director and found myself sitting before our superintendent of curriculum and instruction.
I can't imagine the meeting going better. It was great to see someone with 40+ years in education saying that we need to look at what we do and why we do it. Many of the questions I had, she had as well. One thing we knew for sure was that this vision is fluid and we are in the process of creating it. Questions are encouraged. Push back is needed. Transparency is critical.
Lots of rope. Hope I don't hang myself with it.
Saturday, June 12, 2010
What Else Ya Got?
I know that there are many who are questioning whether or not to make the jump to standards based grading for whatever reason. But the more I think about my own children's education, the more I realize that anything else is crap. My wife and I are probably going to homeschool our boys next year and you know what our main question is? What do we want them to learn?
That's it.
Grades? Nope.
Due dates? Nope.
Packets? Heck no!
Homework? That'd be kinda redundant.
Finish when you finish. Learn when you learn.
Sure, in our classrooms, we have to teach things because we have deadlines imposed upon us and we have to work within them. Kids' learning doesn't give a rip about our deadlines.
So when you decide how you're going to do it next year ask yourself this question:
How would you want your own kids to be taught?
That's it.
Grades? Nope.
Due dates? Nope.
Packets? Heck no!
Homework? That'd be kinda redundant.
Finish when you finish. Learn when you learn.
Sure, in our classrooms, we have to teach things because we have deadlines imposed upon us and we have to work within them. Kids' learning doesn't give a rip about our deadlines.
So when you decide how you're going to do it next year ask yourself this question:
How would you want your own kids to be taught?
Friday, June 11, 2010
Deal With It
So what do you do?
My 8 year old wanted to stay behind with my wife and two of his brothers to do some more shopping while I took the rest of the clan home. As we were leaving the parking lot of our local Costco, Nevan changed his mind. He wanted to go with Dad. Alright, so we made the quick switch and we went our separate ways. Unfortunately, Nevan thought twice about his decision but it was too late. I had frozen foods and a 35 minute drive ahead, so, tough luck son.
Then came the meltdown. And what a meltdown it was.
I've seen so many parents use the redirect with a kid having a meltdown. Make 'em think about something else and the behavior changes. Seems simple enough, but the problem with that is that the kid learns nothing. It's no more than behavior modification via manipulation. Yeah, I don't usually take that route.
I think it's important for my son to learn how his thinking is selfish. Can he be disappointed? Sure. But does he need to make everyone else pay for it? No way.
So we have a conversation about how whining doesn't do anything to change things. We talk about how he was allowed to make a decision and now he has to deal with the consequences of his decision--for better or worse. We discuss how it isn't fair to the others around him for him to carry on as he was. Sure you're disappointed. Sure you're big brown eyes well up with those crocodile tears which make me, for a moment, reconsider the importance of getting the bag of frozen berries home before they melt. But the meltdown has to stop.
Would it have been easier to redirect? Yeah, but it would have been a temporary fix. I want my kids to grow up being able to take their thoughts captive and keep them from becoming behaviors. I don't want to paint over the cracks in my kids character; I want to fix the foundation. That takes time. Lots of time.
So does teaching. When you see a kid doing a procedure incorrectly, do you just show them the right way or even worse (and if you do this, please stop) take the pencil out of their hands and do it for them? Or if you are discussing a concept and a student demonstrates a misconception, do you simply correct it or do you ask questions to get them to see the error of their thinking?
Good teaching takes time. Patience. Relationships.
Everything else is just painting cracks.
My 8 year old wanted to stay behind with my wife and two of his brothers to do some more shopping while I took the rest of the clan home. As we were leaving the parking lot of our local Costco, Nevan changed his mind. He wanted to go with Dad. Alright, so we made the quick switch and we went our separate ways. Unfortunately, Nevan thought twice about his decision but it was too late. I had frozen foods and a 35 minute drive ahead, so, tough luck son.
Then came the meltdown. And what a meltdown it was.
I've seen so many parents use the redirect with a kid having a meltdown. Make 'em think about something else and the behavior changes. Seems simple enough, but the problem with that is that the kid learns nothing. It's no more than behavior modification via manipulation. Yeah, I don't usually take that route.
I think it's important for my son to learn how his thinking is selfish. Can he be disappointed? Sure. But does he need to make everyone else pay for it? No way.
So we have a conversation about how whining doesn't do anything to change things. We talk about how he was allowed to make a decision and now he has to deal with the consequences of his decision--for better or worse. We discuss how it isn't fair to the others around him for him to carry on as he was. Sure you're disappointed. Sure you're big brown eyes well up with those crocodile tears which make me, for a moment, reconsider the importance of getting the bag of frozen berries home before they melt. But the meltdown has to stop.
Would it have been easier to redirect? Yeah, but it would have been a temporary fix. I want my kids to grow up being able to take their thoughts captive and keep them from becoming behaviors. I don't want to paint over the cracks in my kids character; I want to fix the foundation. That takes time. Lots of time.
So does teaching. When you see a kid doing a procedure incorrectly, do you just show them the right way or even worse (and if you do this, please stop) take the pencil out of their hands and do it for them? Or if you are discussing a concept and a student demonstrates a misconception, do you simply correct it or do you ask questions to get them to see the error of their thinking?
Good teaching takes time. Patience. Relationships.
Everything else is just painting cracks.
Thursday, June 3, 2010
Student Creations
Last year, my kids blew me away with this. This year, I was a little more prepared for what we might be able to do with projectile motion. We spent quite a bit of time on vertical motion as part of our standard curriculum, but once we finished with our required standards, we turned our focus towards trig ratios and applying them to motion problems. I built a few applets using GeoGebra to help my students visualize the motion and it sparked an end of year project that these kids are really proud of.
Abel, Matt and Robert
These kids were the first to figure out how to model the projectile. They used the rest of their time trying to dial in the effects. We couldn't figure out how to make the backgrounds of pictures transparent, so they spent a bunch of time defining polygons to cover the white areas. The definitions were really tricky because they had to be defined in terms of the point that was being projected otherwise the image would move but the polygon would remain static.
Check their applet here.
David, Jett and Sartaj
This group really spent some time dialing in their applet. In my opinion, it's probably the most aesthetically pleasing.
Check their applet here.
Sierra, Brandon H. and Brandon J.
The tricky part of this applet was in defining the condition to display the "Bullseye!" text. Since the center of the board is an ellipse (to establish a perspective) these students had to define four points to represent the vertical and horizontal extremes of the ellipse. They then had to determine a set of inequalities which would describe when the point of the dart actually fell within the range of those four points.
Check their applet here.
Marco, Brandon M. and Lazaro
The thing I really like about this applet is how careful they were with their facts. The fence height can change from 3' (Dodger Stadium left/right field) to 37' (Fenway Park's Green Monster). They had to define many points in terms of other points in order to get the fence to be dynamic.
Check their applet here.
Fareen, Alec and Breanna
This group took this project by the horns, big time. They tackled two different motion problems in one. They have a projectile and the bird flies in a linear path defined by an angular velocity. They ran into a snag because their scale was so large that the applet ran incredibly slow. So they spent some time tweaking the axes in order to end up with a really cool applet.
Hit the duck and you'll see their sense of humor--trust me.
Check their applet here.
Jodie, Abraham and Destin
This group had a HUGE vision for this project. They wanted the pitch to come in as a projectile and then leave the batter with a greater angle and greater velocity. The timing on this was difficult at best. They managed to get two projectiles occuring at different times, but had to adjust the time slider to do so. There were times that this one stumped me. I really appreciated the challenge they took on.
Check their applet here.
Creston, Mackay, Jared and Alex
Let's blow up a castle. What else can you say? This group really paid attention to detail. Heck, they even made the clouds move. Hit the castle and get a mushroom cloud. What's not to like about that?!
Check their applet here.
Frankie, Alex and Dil
If you knew these guys, you'd see how appropriate a flying monkey is to their applet. Again, with the details. Determining the condition to show the final image took some time. How close does the monkey have to get to the target in order for the launch to be a success? They mulled it over and drew some strong conclusions.
Check their applet here.
My Role
I asked a lot of questions. Direct instruction was necessary on things specific to GeoGebra like the coordinates of point B can be understood as (x(B),y(B)) but nearly all of the manipulation of the equations was done by them. If a group got stuck on how to make the animation end, the standard line of questioning would go something like:
"What do you want the applet to look like when the animation ends?"
"In order to get that result are we more interested in the height of your projectile or the distance?"
"How can we describe the height of a projectile?" or "How can we describe the distance it's travelled?"
Once they were able determine which model they needed to use (vertical motion or linear motion), we'd set up the equation. A lot of them looked something like:
h = -16t2 + v sin(α) t + s or d = v cos(α) t
and they'd play with it until they solved for t. Sometimes we'd have to think of the velocity in terms of something times t and go back to the original equations h = -16t2 + vt + s or D = rt in order for them to realize that v sin(α) and v cos(α) are just rates.
I don't think I've had more fun over a two week period in the classroom. Ever.
The best part was when the groups would finally export their applet to .html and then we'd go back to my desk where I showed them how to replace the current code with the animation code. The looks on their faces when they saw something they had created actually do what it was supposed to do was priceless.
Yeah, we'll prolly do something like this again next year.
Tell 'em what you think in the comments.
(Note: I had planned on having students write their reflections and link to their projects on our class blog. However, due to a time crunch at the end, I've posted them all here. They'll be checking this post for your feedback.)
Wednesday, June 2, 2010
Let 'em Play
Sometimes you just have to cut the kids loose and see what they come up with. The year's winding down and I have kids reassessing (today's the last day) like crazy. But some kids are proficient on all of our skills so I gave them a short GeoGebra lab on Drawing vs. Construction. A student came over and showed me this:
The little boogers were playing around with sliders, defined 'a' as a variable, plugged in the equation:
y = x2 + a
and proceeded to create any variation of that equation they could find. Opposite functions, inverse functions, opposite inverses, etc.
They marveled at the symmetry and how the whole picture changed as they adjusted the slider 'a.'
I've had kids each year ask, "How do you make a parabola lie on its side?"
I've always responded with, "I don't know. Let's see what we can figure out."
No one's ever pursued it.
These kids discovered it by "playing."
Tuesday, June 1, 2010
You Don't Prune Cotton!
My opinion of projects has changed. I used to look for something that encompassed as many skills as possible as an alternative way for students to demonstrate their ability to perform a process. I'm over that.
This year's Farming Project went better than expected. Last year, I assigned it to groups, but this year I had each student choose a different number of acres and go to work. Instead of worrying about all the how to's, I focused on they why's. I didn't realize how conceptually strong this project could be until I started having conversations with students as they had each task signed off. Kids had to discern that you don't prune cotton, you don't spray herbicide on acres you're not farming and you can't hire 14.3 crews. Finding the graphs of the inequalities was the easy part so I had them explain to me what each part of the expressions meant within the context of the problem.
Learning truly became a conversation.
The most interesting part came when I had students do a self assessment. I had already spoken with each student regarding each task so I knew what they knew. I was interested in how much they thought they knew. I think that by the time we tackle a project like this, their own feedback becomes more important than mine.
What do you think you know?
Each student assessed themselves in the following categories:
Surprisingly (or not) each student gave a very accurate (and honest) self assessment. My opinion became incidental.
I asked what they learned.
Highlights:
Reflection
This year's Farming Project went better than expected. Last year, I assigned it to groups, but this year I had each student choose a different number of acres and go to work. Instead of worrying about all the how to's, I focused on they why's. I didn't realize how conceptually strong this project could be until I started having conversations with students as they had each task signed off. Kids had to discern that you don't prune cotton, you don't spray herbicide on acres you're not farming and you can't hire 14.3 crews. Finding the graphs of the inequalities was the easy part so I had them explain to me what each part of the expressions meant within the context of the problem.
Learning truly became a conversation.
The most interesting part came when I had students do a self assessment. I had already spoken with each student regarding each task so I knew what they knew. I was interested in how much they thought they knew. I think that by the time we tackle a project like this, their own feedback becomes more important than mine.
What do you think you know?
Each student assessed themselves in the following categories:
- Time management
- Understanding the math behind the project
- Explaining the math behind the project
- Ability to work independently (how much help did you need?)
- Overall performance
Surprisingly (or not) each student gave a very accurate (and honest) self assessment. My opinion became incidental.
I asked what they learned.
Highlights:
- I learned that all of these standards and things we have learned have a place in the real world. I will actually use the things I have learned in eighth grade.
- I learned how you could use the math we're learning in class in a real life situation.
- I also learned how to manage my time a little better.
- I learned how we can take our math skills and apply them to everyday situations. I also learned how to do Standard 15.0 a little better because of Task 8 when we had to combine the 3 workers' times.
- I definitely learned how to use geogebra better like on project #4 for example. I thought it was so cool to see how the intersection of the two maximum profit lines gave me the acreage of crops I'm planting. I rediscovered how to do a lot of skills I've already learned and learned how to apply them better like [task] #7 [standard]15.0.
- I learned that math can actually be applied to the real world! I learned how to do a mixture problem a bit differently than i originally knew how before doing this project.I ACTUALLY HAD FUN WITH THIS!! :)
- I now understand some math skills A LOT better, doing this. I definitely am better at standard 15.0, that killer..:) But overall, I just understand a lot of things better now.
- I learned how to finally do the mixture problems and how to do the work problems from standard 15.0.
- I learned so much more about how math is used in the real world. [YEAH, THANKS MR.COX FOR RUINING MY LIFE AND TURNING EVERYTHING I SEE INTO A MATH PROBLEM.] just kidding. :-)But seriously, after this project, I really did see math in the real world a lot more. I also had fun practicing skills I hadn't used in a while. Most of these skills I already knew, but 15.0 was a real tough one, that I finally mastered! [for the most part..]
Reflection
- Next year I'm going to be less specific with my directions. Telling them to put the inequalities into a polygon takes away some of the fun in discovering why we graph them. We will have already done some linear programming, so I shouldn't have to explicitly tell them to do it. I realize that some kids will just piggyback on the work of others, but I'll usually catch that once they have to justify their answers to me.
- Assign due dates. Even if I don't hold to them, having a flexible schedule will help students manage time better.
- Focus on the concepts. They've been tested on skills to death. The last thing they need is another project that asks for skill after skill with no real understanding of why the skill applies in a particular situation.
- Get rid of having students determine the equation of one of the property lines. It's completely contrived and has no real application to the project.
- Grade for complexity of property shape. Students determine their acreage before they have a plot of land. Having them determine the dimensions of the property to fit the acreage becomes way more interesting if a student chooses a pentagon or hexagon (as opposed to a triangle) and they should be rewarded for taking a stab at it.









