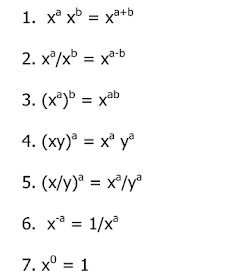Yesterday, Dan threw out this tweet:
So, naturally, I had to try this:
If I were using this in class, I'd probably animate it so students had to choose values for velocity, start height and distance and hit "GO."
Download original .ggb file.
Pages
▼
Thursday, December 30, 2010
Tuesday, December 21, 2010
Adventures in Pedagogy: Number Sense
Nevan: Dad when do you have to go back to work?
Me: I'm off for three weeks.
Nevan: Ok. Well, today's the 20th. Plus 7, that's the 27th. 4 more to the 31st. The other 3 for January 3rd. Plus 7 more...the 10th. You go back to work on the 10th.
- Posted from my iPhone
Me: I'm off for three weeks.
Nevan: Ok. Well, today's the 20th. Plus 7, that's the 27th. 4 more to the 31st. The other 3 for January 3rd. Plus 7 more...the 10th. You go back to work on the 10th.
- Posted from my iPhone
Friday, December 17, 2010
Volume
We had a few presentations to watch due to absences and one in particular sparked a pretty good discussion. One, in particular, focused on how to find the volume of a cylinder. The presenter told the class how to find the volume and then proceeded to explain why we find the volume of a cylinder by using the formula:
But she didn't stop there. She asked herself and the class:
Why is that the formula?
She went on to describe how it's like taking a bunch of CDs and stacking them. We know we can find the area of a CD.
That's when things got interesting. I heard comments like these:
V = πr2h
But she didn't stop there. She asked herself and the class:
Why is that the formula?
She went on to describe how it's like taking a bunch of CDs and stacking them. We know we can find the area of a CD.
That's when things got interesting. I heard comments like these:
- We use the height to determine how many circles it takes to make up the cylinder.
- But it's just like a line [it has no height]. How do you get volume with that?
- If you have a thing of CDs and stack them...you're going from 2D to 3D.
- But area has no height. We're stacking things that have no height.
So we talked a little about finding the volume of a rectangular prism is just counting the number of cubic units it takes to fill the prism and how doing that with a cylinder is tough because its round. I liked the direction they were going with this by trying to talk about something larger by defining it by its smaller parts. So small, in fact, that the height is practically 0.
I ask, So how can you find the volume of a sheet of paper?
A student takes a sheet of paper puts a ruler up to it as if he's going to measure the height of one sheet of paper and one of his group members says:
Dude, why don't we just make a stack that's 1" tall and divide by the number of sheets it took to make it?
They all agreed that would work and I thought we were ready to move on when a boy asks, "So what's the volume for a sphere?"
They wrestle with this for a minute or two when one of them turns to me and says, "Mr. Cox, you know, huh?"
Yep.
But you're not going to tell us are you?
Nope.
A student opens his planner to the Math Formulas page and starts to tell us what the formula is when one says,
I don't care what the formula is. I want to know why that's the formula.
Next semester's gonna be fun.
Thursday, December 16, 2010
Exterior Angles
"Hey, Mr. Cox. Is there a theorem that says this?"
I'm not sure. Why do you think that's true?
"Well if a triangle has 180o and I know two of them, then the third one has to be whatever's left over from 180o. But that third angle and x make a straight line so they have to add up to 180o too."
If it's true, what would you call it?
"I remember seeing something about 'exterior angle' in the index one time, so that's taken. Maybe I'll look it up and see what that one means."
*goes and looks up Exterior Angle Theorem*
"Ah, man! Someone already discovered it."
I'm not sure. Why do you think that's true?
"Well if a triangle has 180o and I know two of them, then the third one has to be whatever's left over from 180o. But that third angle and x make a straight line so they have to add up to 180o too."
If it's true, what would you call it?
"I remember seeing something about 'exterior angle' in the index one time, so that's taken. Maybe I'll look it up and see what that one means."
*goes and looks up Exterior Angle Theorem*
"Ah, man! Someone already discovered it."
Wednesday, December 15, 2010
Download Time
I kept reading about all the fun everyone was having with the ProjectEuler thing, so I thought I'd give it a try. I thought it was pretty easy until I got to that one problem where they ask you to add up all the multiples of 3 or 5 that are less than 1000. I hear that people are using Python and letting the computer do the number crunching.
So, I think to myself, "Self, you took Pascal about 20 years ago back when RAM was measured in single digits and you said 'bless you' if someone said, 'gigahertz.' in college and absolutely hated that freaking class came out a better person because of it. This may be something you don't have time for, I mean you have 5 kids. Wake the heck up an enriching experience. And besides...Pascal...Python, they both start with Ps yeah, that's probably the only thing they have in common. You got this, man. G'head, give it a try."
I go to the Python site and read every thing I can to figure out which version would best suit me. (There's a lot to choose from, you know.) I can't find anything anywhere. It's all so confusing. Please. Someone. Give me a nudge in the right direction.
So I take a shot in the dark and go with v.2.7.
Anyway, this isn't really about Python. It's about the video I grabbed while downloading the program. I'm going to have my kids wrestle with it after break.
I may not ever find the solution to that really difficult problem about multiples of 3 and 5, but at least I got a lesson out of it.
Rates of Change
Sometimes we just stumble onto stuff. I've taught slope intercept form for a lot of years, but the more I try to stay true to the concept, the more my students demonstrate innovative ways of seeing things. We just finished up linear equations and their graphs. The equations part of things usually culminates with a student taking two points and writing the standard form of the equation by way of this process:
Step 1: Find slope
Step 2: Write in point slope form
Step 3: Solve for y to get slope-intercept form
Step 4: Rewrite in Standard Form
I've usually encouraged this process as it seemed to be the efficient way to cover the majority of these skills. But this year, I kept it much more loose. We all agreed that if the two points given define a line with an integer for the y-intercept, then it's pretty easy to write in slope intercept form.
I mean, c'mon, we've got the slope and we see the y-intercept plain as day.
But what happens here?
We know the slope is 1/7 and we can estimate the y-intercept, but how can we know the y-intercept?
Rate of Change
Most of the time when we discuss Slope we talk about the path from one lattice point to another. For example, the slope between A and B is 1/7 because we go up 1 and over 7 to get from A to B.
But we have been doing more with rate of change as a unit rate which means that for every 1 unit of horizontal change, we increase 1/7. Yeah, I know that sounds elementary, but it makes a difference in how your kids see these things.
If each step increases 1/7, we increase a total of 4/7 to get to the y axis. Therefore, y-intercept is 4 4/7. We still talk about point-slope form, but this beats the heck out of the plug and chug that usually goes along with it.
Step 1: Find slope
Step 2: Write in point slope form
Step 3: Solve for y to get slope-intercept form
Step 4: Rewrite in Standard Form
I've usually encouraged this process as it seemed to be the efficient way to cover the majority of these skills. But this year, I kept it much more loose. We all agreed that if the two points given define a line with an integer for the y-intercept, then it's pretty easy to write in slope intercept form.
I mean, c'mon, we've got the slope and we see the y-intercept plain as day.
But what happens here?
Rate of Change
Most of the time when we discuss Slope we talk about the path from one lattice point to another. For example, the slope between A and B is 1/7 because we go up 1 and over 7 to get from A to B.
But we have been doing more with rate of change as a unit rate which means that for every 1 unit of horizontal change, we increase 1/7. Yeah, I know that sounds elementary, but it makes a difference in how your kids see these things.
If each step increases 1/7, we increase a total of 4/7 to get to the y axis. Therefore, y-intercept is 4 4/7. We still talk about point-slope form, but this beats the heck out of the plug and chug that usually goes along with it.
Tuesday, December 14, 2010
Semester Projects
I'm still a believer in the taxonomy I wrote about a while back. I can teach duplication like no body's business and finding ways to get students to apply what we are learning isn't a problem. It's the creation part that I get hung up on. I want my students to find ways to make their own connections with the math.
Our attempt at creation.
Assignment: Pick something that interests you; something that you can connect to one of the concepts we've covered this semester. Learn as much as you can about it. Tell us what you've learned.
I only had one requirement: Don't ask yourself, "What is Mr. Cox looking for?"
Keep in mind that I get the kids who taught Clever Hans how to read people. They are fantastic kids, but they also have learned how to game the system. Find a way to jump through the fewest hoops (or at least the most efficient way to jump through them all) while gathering as many points as possible on the way to be-all-end-all of educational existence: The A.
This assignment scared the crap out of some of them. This was the most unhelpful I've ever been. I allowed them to work on their own or in groups (up to 3). We discussed the benefits and pitfalls to working alone vs. working with a group.
We discussed what type of media would best serve their presentation. I learned that when I say, "presentation," kids hear "PowerPoint." I told them that it didn't matter what they used to convey their learning, but if they were going to use visuals, they needed to work with their content, not against it. Does that mean I talked to them about design? I guess I've come a long way since this comment. (Full comment here.)
As we approached the due date, we started to discuss how this assignment should be graded. Again, I took a back seat on this. We just recently opened up the sequoiahawks.org domain for our students to gain access to Google Apps, so this was a great opportunity to have them work collaboratively on a rubric. My 8th graders had some experience with this as we used G-Docs last year, but this was new to all of my 7th graders. The only input I had in this process was to create the document and ask a few questions. The rest was all them.
I found that some of the conversations that took place regarding what to include, what to exclude and what categories were the same (ie. "Aren't self interest and effort the same thing?") were very refreshing. These kids were really thinking about what was important.
The Presentations
It was nice to see students take things that we have done in class and either find a context for them or extend them beyond what we've done in class. There were a couple of presentations that stood out for their creativity and some for their thought provoking qualities. We had a few nice class conversations that came from questions posed in the presentations. Both classes had someone ask the question: is .999...=1? which provided a great opportunity for discussion. The 8th graders were pretty curious and this led to a conversation on ∑ notation, ∞ and limits.
We did have a few groups that mailed in the projects, though. I had a tough time not airing them out publicly (in one case I did) as they showed complete disrespect for their classmates' time. This demonstrates that the hoop jumping is not quite out of their system, although I'd say we are off to a decent start.
Our attempt at creation.
Assignment: Pick something that interests you; something that you can connect to one of the concepts we've covered this semester. Learn as much as you can about it. Tell us what you've learned.
I only had one requirement: Don't ask yourself, "What is Mr. Cox looking for?"
Keep in mind that I get the kids who taught Clever Hans how to read people. They are fantastic kids, but they also have learned how to game the system. Find a way to jump through the fewest hoops (or at least the most efficient way to jump through them all) while gathering as many points as possible on the way to be-all-end-all of educational existence: The A.
This assignment scared the crap out of some of them. This was the most unhelpful I've ever been. I allowed them to work on their own or in groups (up to 3). We discussed the benefits and pitfalls to working alone vs. working with a group.
We discussed what type of media would best serve their presentation. I learned that when I say, "presentation," kids hear "PowerPoint." I told them that it didn't matter what they used to convey their learning, but if they were going to use visuals, they needed to work with their content, not against it. Does that mean I talked to them about design? I guess I've come a long way since this comment. (Full comment here.)
As we approached the due date, we started to discuss how this assignment should be graded. Again, I took a back seat on this. We just recently opened up the sequoiahawks.org domain for our students to gain access to Google Apps, so this was a great opportunity to have them work collaboratively on a rubric. My 8th graders had some experience with this as we used G-Docs last year, but this was new to all of my 7th graders. The only input I had in this process was to create the document and ask a few questions. The rest was all them.
I found that some of the conversations that took place regarding what to include, what to exclude and what categories were the same (ie. "Aren't self interest and effort the same thing?") were very refreshing. These kids were really thinking about what was important.
The Presentations
It was nice to see students take things that we have done in class and either find a context for them or extend them beyond what we've done in class. There were a couple of presentations that stood out for their creativity and some for their thought provoking qualities. We had a few nice class conversations that came from questions posed in the presentations. Both classes had someone ask the question: is .999...=1? which provided a great opportunity for discussion. The 8th graders were pretty curious and this led to a conversation on ∑ notation, ∞ and limits.
We did have a few groups that mailed in the projects, though. I had a tough time not airing them out publicly (in one case I did) as they showed complete disrespect for their classmates' time. This demonstrates that the hoop jumping is not quite out of their system, although I'd say we are off to a decent start.
Friday, December 3, 2010
Thursday, December 2, 2010
Ticket Prices
The other night, Dawson and I were doing some math together and we ran across a problem that asked to interpret a scatterplot for the average movie ticket prices for the past 10 years. We poked around online until we found this image:
 |
We used the information to come up with an average price and dropped it into Excel and came up with a best fit line and all. Personally, I find Excel clunky, but since we could use it to quickly calculate average price, we went with it. But it just so happens that I'm doing linear relationships with my 7th graders, so I get a two-fer with this one. Step 1: Calculate average ticket prices and created a scatter plot. This went pretty quickly, but one group mistook the raw number for the average ticket price. For example: from 2003 to 2004 the revenue went down, but so did the number of tickets sold. The raw numbers led this group to believe that the prices went down. After a quick conversation, they realized that if the number of tickets sold decreases as well, the price can actually increase. Step 2: Predict the price of a ticket in 2020 and justify answer. Most groups used the trend of the graph to predict, but one group actually calculated the average rate of change from year to year and came up with $.24/year. They used this to extrapolate a price in 2020. Step 3: Decide what type of curve best fits the data. Two camps on this one: Those who thought it was linear and those who thought it would be like "the graph we got when we did compound interest." Nice work kids. Note: We will explore that exponential thingy but we ran out of time today. Step 4: What line would best fit the data? They were creating a group graph so they took a meter stick and just plopped it down where they thought the line should go. Step 5: Estimate the equation of that line. We played with this applet yesterday, so students had a pretty good idea how to use the graph to predict what the equation should look like. I was pleasantly surprised at the fact that all groups decided the rate of change was somewhere between $.22 and $.25 and all said the initial condition was $4.34. Step 6: Let GeoGebra work her magic. Add the ordered pairs and use the "Best Fit Line" tool to calculate the regression. Step 7: How good was our guess? |
Tuesday, November 30, 2010
All Present and Accounted For
Finally, everything from the old blog is here. The process was actually pretty simple:
1. Export posts from Wordpress to XML. I had to do this in two increments because the conversion site only works for files smaller than 1MB.
2. Convert the XML using Wordpress2Blogger.
3. Import the converted XML to Blogger.
Done!
1. Export posts from Wordpress to XML. I had to do this in two increments because the conversion site only works for files smaller than 1MB.
2. Convert the XML using Wordpress2Blogger.
3. Import the converted XML to Blogger.
Done!
Now What?
Paul Lockhart:
Don't be too quick to write this off as impossible given our current system.
In particular, you can’t teach teaching. Schools of education are a complete crock. Oh, you can take classes in early childhood development and whatnot, and you can be trained to use a blackboard “effectively” and to prepare an organized “lesson plan” (which, by the way, insures that your lesson will be planned, and therefore false), but you will never be a real teacher if you are unwilling to be a real person. Teaching means openness and honesty, an ability to share excitement, and a love of learning. Without these, all the education degrees in the world won’t help you, and with them they are completely unnecessary.
Don't be too quick to write this off as impossible given our current system.
Saturday, November 27, 2010
Insomnia
I went to bed with this problem swimming around in my mind. I kept trying to figure out how to construct in GeoGebra. I told it to be quiet so I could sleep. It wouldn't.
Problem:
Double click to open in a new window.
It's almost 1am. I'm going to bed now.
Problem:
Given two squares, deconstruct and reconstruct as one square.
Double click to open in a new window.
It's almost 1am. I'm going to bed now.
Friday, November 26, 2010
I Love This Problem
I love simple problems with simple proofs. And I love GeoGebra even more for making it possible for my students to use their induction to help the proof along.
Find the shortest path from point C to point D that touches line AB.1
1 This problem is one of the many reasons I'm glad I finally got around to reading this.
Problem:
Find the shortest path from point C to point D that touches line AB.1
1 This problem is one of the many reasons I'm glad I finally got around to reading this.
Monday, November 8, 2010
Video Assessment
I mentioned a while back that I thought a Flip camera may be a pretty decent assessment tool. Today, I had one group having a great discussion about distilling the process for finding a perpendicular bisector into a few steps. I grabbed the Flip and sat down with them. Forgive me if you can't read their steps very well, I'm still working out the kinks.
Perpendicular Bisector from David Cox on Vimeo.
I think I see some real potential here. Kids can record themselves and then we can go to After Effects and add some stills of their work. I don't know, maybe I'm getting ahead of myself. Feedbackwelcome necessary, please.
Perpendicular Bisector from David Cox on Vimeo.
I think I see some real potential here. Kids can record themselves and then we can go to After Effects and add some stills of their work. I don't know, maybe I'm getting ahead of myself. Feedback
Thursday, November 4, 2010
WCYDWT: Projectiles
The camera angle messes with the perspective a bit, but I still find these images interesting. Does it mess with the perspective so much that it ruins the problem or does it just lead to another discussion on how things aren't always as they seem. I'm thinking that I'll show the video, but make digital and hard copies of the photos available to students.
Question #1
Projectile Question (YG) from David Cox on Vimeo.
Question #2
Projectile Question (PG) from David Cox on Vimeo.
Answer #1
Projectile Answer (YG) from David Cox on Vimeo.
Answer #2
Projectile Answer (PG) from David Cox on Vimeo.
Next up:
Create something that helps kids see vertical and horizontal motion independence.
Question #1
Projectile Question (YG) from David Cox on Vimeo.
Question #2
Projectile Question (PG) from David Cox on Vimeo.
Answer #1
Projectile Answer (YG) from David Cox on Vimeo.
Answer #2
Projectile Answer (PG) from David Cox on Vimeo.
Next up:
Create something that helps kids see vertical and horizontal motion independence.
Wednesday, November 3, 2010
Friday, October 29, 2010
Toaster Remix
Dan's getting more mileage out of this problem than I am. Either way, there are some good questions being asked re: pseudocontext, relevancy and so on. So I'm curious, which one would you use and why?
(Note: don't check the answer until you've done the regression. It's much more fun that way.)
The Original (setting 1,2 5 and 7)
Toaster Question from David Cox on Vimeo.
Or this?
Toaster Question (1-4) from David Cox on Vimeo.
Or this?
Toaster Question 2 (1-4) from David Cox on Vimeo.
The Answer
Toaster Answer from David Cox on Vimeo.
(Note: don't check the answer until you've done the regression. It's much more fun that way.)
The Original (setting 1,2 5 and 7)
Toaster Question from David Cox on Vimeo.
Or this?
Toaster Question (1-4) from David Cox on Vimeo.
Or this?
Toaster Question 2 (1-4) from David Cox on Vimeo.
The Answer
Toaster Answer from David Cox on Vimeo.
Wednesday, October 27, 2010
WCYDWT: Toast
Funny what you come up with when making breakfast for your kids.
Toaster Question from David Cox on Vimeo.
Wednesday, October 20, 2010
Crushing
Between 2nd and 3rd periods I usually step outside class and wander the plaza as kids are heading to their next classes. Today I happened upon a group of students who were waiting for their teacher—many of whom are in my 4th/5th block.
So I take a seat next one of my students as he's waiting for the door to be unlocked and we discuss how his faux-hawk could have been used to cut the stress joints in the concrete slab beneath our feet, you know, because that's just me.
So he turns to me and says, "You know, Mr. Cox, you're my only teacher who I could see talking to me outside of class."
Wait! What? Why?
"I dunno, other teachers just don't talk to me outside of class."
Don't they know who you are? They're missing out.
So I take a seat next one of my students as he's waiting for the door to be unlocked and we discuss how his faux-hawk could have been used to cut the stress joints in the concrete slab beneath our feet, you know, because that's just me.
So he turns to me and says, "You know, Mr. Cox, you're my only teacher who I could see talking to me outside of class."
Wait! What? Why?
"I dunno, other teachers just don't talk to me outside of class."
Don't they know who you are? They're missing out.
Wednesday, October 6, 2010
Now We're Cookin'
We were going over our quizzes yesterday and I had a student make an interesting observation about the rubric we use to determine the overall score on a concept/skill.
5: Strong concept; No errors
4: Strong concept; Errors present
3: Flawed concept; No errors
2: Flawed concept; Errors present
1: Little or no attempt
She says, "This reminds me of the first time my brother and I made waffles without my dad helping us."
Oh yeah, what happened?
"We used baking soda instead of baking powder and they turned out really bad."
How's that like our quiz?
"We can know what we are doing but make a simple error and it messes the whole problem up."
So what would you rather do: know how to cook the waffles and use the wrong ingredients or use the right ingredients but not know how to cook?
"I'd rather make the mistake with the ingredients."
Why?
"Because all I have to do is use the right stuff and the waffles would've been good."
Is that easier to correct than if you had all the right stuff but you didn't know how to cook?
"Yeah. A lot easier."
5: Strong concept; No errors
4: Strong concept; Errors present
3: Flawed concept; No errors
2: Flawed concept; Errors present
1: Little or no attempt
She says, "This reminds me of the first time my brother and I made waffles without my dad helping us."
Oh yeah, what happened?
"We used baking soda instead of baking powder and they turned out really bad."
How's that like our quiz?
"We can know what we are doing but make a simple error and it messes the whole problem up."
So what would you rather do: know how to cook the waffles and use the wrong ingredients or use the right ingredients but not know how to cook?
"I'd rather make the mistake with the ingredients."
Why?
"Because all I have to do is use the right stuff and the waffles would've been good."
Is that easier to correct than if you had all the right stuff but you didn't know how to cook?
"Yeah. A lot easier."
Monday, October 4, 2010
Adventures in Pedagogy: Context Clues
Dawson and I are watching an episode of Star Wars: The Clone Wars and the narrator opens with the usual recap of previous happenings. Towards the end, he says something like:
"...and Anakin Skywalker thwarted the nefarious plot."
So I ask Dawson, "What does nefarious mean?"
"What do you mean, Dad?"
What's nefarious mean? The guy just said that Anakin thwarted a nefarious plot.
*he thinks for a second*
"Well, it must be really bad because he was talking about the evil scientist."
It never ceases to amaze me how these teachable moments just fall in my lap. I just have to be paying enough attention to them and take the time to ask a couple of questions.
I wonder how many of them I actually miss, though.
"...and Anakin Skywalker thwarted the nefarious plot."
So I ask Dawson, "What does nefarious mean?"
"What do you mean, Dad?"
What's nefarious mean? The guy just said that Anakin thwarted a nefarious plot.
*he thinks for a second*
"Well, it must be really bad because he was talking about the evil scientist."
It never ceases to amaze me how these teachable moments just fall in my lap. I just have to be paying enough attention to them and take the time to ask a couple of questions.
I wonder how many of them I actually miss, though.
Sunday, October 3, 2010
Adventures in Pedagogy: Order of Operations
Nevan (8) goes to the counter to grab a pack of gum from a box that contains four different flavors. He says, "Hey Dad, look. There's four different kinds of gum in here."
Oh, yeah? How many of each kind are there?
"Four."
Then how many packs of gum are in the box?
"Ten."
Really? How do you get ten?
"Yeah, we've eaten two."
Oh, yeah? How many of each kind are there?
"Four."
Then how many packs of gum are in the box?
"Ten."
Really? How do you get ten?
"Yeah, we've eaten two."
Saturday, October 2, 2010
Adventures in Pedagogy: Syllogism and Detachment
On our way home Aidan (5) asks, "Dad does it hurt Jesus' hands when we drive?"
Wha?
"Does it hurt Jesus' hands when we drive?"
What do you mean?
"Our truck drives on tires."
Okay.
"And the tires have pokey things." (read: tread)
Right.
"And the pokey things hurt the road."
Yeah, kind of, I guess.
"And Jesus' hands are under the road holding it."
Why do you say that?
Dawson (12) says, "Dad, I think he thinking of 'He's got the whole world...in His hands...'"
Is that what you mean, Aidan?
"Yeah. So then, when we drive, it hurts Jesus' hands, right?"
Wha?
"Does it hurt Jesus' hands when we drive?"
What do you mean?
"Our truck drives on tires."
Okay.
"And the tires have pokey things." (read: tread)
Right.
"And the pokey things hurt the road."
Yeah, kind of, I guess.
"And Jesus' hands are under the road holding it."
Why do you say that?
Dawson (12) says, "Dad, I think he thinking of 'He's got the whole world...in His hands...'"
Is that what you mean, Aidan?
"Yeah. So then, when we drive, it hurts Jesus' hands, right?"
Thursday, September 30, 2010
A Conversation Worth Having
I have a student who is very creative with her math. She just tries stuff, learns from mistakes and constructs her own understanding. It's pretty cool to watch. Today, she was describing to the class how she came up with the standard form of a linear equation we were playing with yesterday by using the slope of the line.
M.M. pipes up, "I don't get how she did that."
What he meant was, "Yeah, I see how that equation works, but I have no idea how she thought of doing that."
She follows her intuition and sees where it takes her.
I told M.M., "I'm not so sure that you really need to know how she came up with it any more than you need to know how an artist paints or how an author writes. What you need to appreciate is the fact that she created some understanding on her own."
I launched into a quick discussion on Duplication, Application and Creation and how many "advanced" kids actually live in the duplication stage, but they just do it faster than everyone else. I can teach duplication and provide opportunity for application, but creation is all them.
Here's what went down as a result.
I.N. : Mr. Cox, your class is the only one I do my homework in.
Why?
I.N. : Because you don't make us do it. Like at home, if my mom makes me go out for a sport, I don't really try because I feel like I'm forced to do it, but I'll go play sports on my own with friends or even with random people. I want to do my homework in here.
J.T.: Actually, Mr. Cox, you don't really force anything on us.
Try not to, anyway.
The conversation went to grades and grading in general and I said I don't really care for grades and wouldn't give them if I didn't have to.
S.N. : Then you need to start your own school, Mr. Cox.
Yeah, that'd be nice but I don't have that kind of money.
L.F.: I'll go work at McDonald's and give you my paycheck.
One kid said, "you're gonna change the system, Mr. Cox" and I kinda let the comment go. But after a few minutes I came back around to it.
You guys really think I'm gonna change the system?
*silence*
"Nah, we're gonna change the system."
M.M. pipes up, "I don't get how she did that."
What he meant was, "Yeah, I see how that equation works, but I have no idea how she thought of doing that."
She follows her intuition and sees where it takes her.
I told M.M., "I'm not so sure that you really need to know how she came up with it any more than you need to know how an artist paints or how an author writes. What you need to appreciate is the fact that she created some understanding on her own."
I launched into a quick discussion on Duplication, Application and Creation and how many "advanced" kids actually live in the duplication stage, but they just do it faster than everyone else. I can teach duplication and provide opportunity for application, but creation is all them.
Here's what went down as a result.
I.N. : Mr. Cox, your class is the only one I do my homework in.
Why?
I.N. : Because you don't make us do it. Like at home, if my mom makes me go out for a sport, I don't really try because I feel like I'm forced to do it, but I'll go play sports on my own with friends or even with random people. I want to do my homework in here.
J.T.: Actually, Mr. Cox, you don't really force anything on us.
Try not to, anyway.
The conversation went to grades and grading in general and I said I don't really care for grades and wouldn't give them if I didn't have to.
S.N. : Then you need to start your own school, Mr. Cox.
Yeah, that'd be nice but I don't have that kind of money.
L.F.: I'll go work at McDonald's and give you my paycheck.
One kid said, "you're gonna change the system, Mr. Cox" and I kinda let the comment go. But after a few minutes I came back around to it.
You guys really think I'm gonna change the system?
*silence*
"Nah, we're gonna change the system."
Tuesday, September 28, 2010
Equations Three Ways
I've never really been satisfied with how I teach students to solve equations. No matter what, it ends up being one big algorithm and kids have no idea why one side of the equation is equal to the other. Here's what I'm doing to try to fix that.
Modeling
Strength: It's not math. It's a puzzle.
Weakness: Dealing with negatives is a real pain in the butt.
Guess and Check
I actually really like this method. Guess and check is probably my most under-used problem solving strategy, but using it to solve equations has been really helpful. I've noticed a greater understanding of rate(s) of change, using information from wrong answers to help find right ones and checking answers--something most kids don't want to do--is embedded in the process.
We've gotten to the point where we can nail the answer on the third guess by using the information gained in the first two--even for equations with non-integer solutions.
Strength: Students understand that simplified expressions on each side of the equal sign end up looking the same every time (ax + b = cx + d). Rate of change is very useful. Being wrong helps you to become right. Did I mention they are checking their answers?
Weakness: Leave 'em in the comments.
From Construction to Deconstruction
We spend a lot of time teaching kids how to break things down whether it's reducing fractions, simplifying radicals or solving equations but we rarely (read: I rarely) have taught them how to construct things that may eventually need to be deconstructed.
Constructing a more complicated equation from a simple equation has helped my students understand that, no kidding, the two expressions on opposite sides of the equal sign are equivalent. I've used the just-unwrap-the-present illustration many times, but we really need to teach the students to wrap one up first. Having them list their steps for construction makes the process for actually solving the equation seem much more natural. When I say, "just use the inverse order of operations" --or whatever completely abstract thing I've been known to throw out there in order to make myself feel better when they keep screwing it up--it makes no sense to them. This helps.
Strength: Kids get a grasp of which operation to tackle first while solving for x.
Weakness: Very complicated equations with variables on both sides don't seem so natural when you begin with x = 2.
I've heard rumors that there are some teachers who actually teach solving equations by graphing. Never seen it in the wild, though.
Modeling
Strength: It's not math. It's a puzzle.
Weakness: Dealing with negatives is a real pain in the butt.
Guess and Check
I actually really like this method. Guess and check is probably my most under-used problem solving strategy, but using it to solve equations has been really helpful. I've noticed a greater understanding of rate(s) of change, using information from wrong answers to help find right ones and checking answers--something most kids don't want to do--is embedded in the process.
We've gotten to the point where we can nail the answer on the third guess by using the information gained in the first two--even for equations with non-integer solutions.
Strength: Students understand that simplified expressions on each side of the equal sign end up looking the same every time (ax + b = cx + d). Rate of change is very useful. Being wrong helps you to become right. Did I mention they are checking their answers?
Weakness: Leave 'em in the comments.
From Construction to Deconstruction
We spend a lot of time teaching kids how to break things down whether it's reducing fractions, simplifying radicals or solving equations but we rarely (read: I rarely) have taught them how to construct things that may eventually need to be deconstructed.
Constructing a more complicated equation from a simple equation has helped my students understand that, no kidding, the two expressions on opposite sides of the equal sign are equivalent. I've used the just-unwrap-the-present illustration many times, but we really need to teach the students to wrap one up first. Having them list their steps for construction makes the process for actually solving the equation seem much more natural. When I say, "just use the inverse order of operations" --or whatever completely abstract thing I've been known to throw out there in order to make myself feel better when they keep screwing it up--it makes no sense to them. This helps.
Strength: Kids get a grasp of which operation to tackle first while solving for x.
Weakness: Very complicated equations with variables on both sides don't seem so natural when you begin with x = 2.
I've heard rumors that there are some teachers who actually teach solving equations by graphing. Never seen it in the wild, though.
Thursday, September 16, 2010
Hijinx
Teaching can be stressful. So, I find it important to loosen things up a little.
Like, maybe, accidentally leaving the guidance office with things that happen to belong on the secretaries' desks only to return them in the form of gifts on Secretary Appreciation Day in the Spring. We all get a good laugh out of it--except that one time a fight almost broke out because Mrs. P thought Ms. A had taken her tape dispenser.
Or, rearranging the furniture in a teacher's room while they're out on school business. (Note: don't do that one again.)
Or dressing up like a colleague on Nerd Day. (Well, I thought it was funny.)
Most of these antics were the result of me being part of a cohort of younger teachers who were now teaching at the same high school we had attended.
Things changed when I moved to the new middle school. Most of the "new" staff had come from the same school, so I had to take some time adjusting to the culture that many had brought with them. Seemed like the right thing to do. As a result, I quickly became good friends with Mr. G, who had come to us by way of a neighboring district. We'd usually end up in one of our rooms by the end of the lunch period. But one day, I walked into his room and he had a visitor. I felt like I had kind of intruded so the next time I walked up there, I knocked as I entered to let him know I was coming. He made the mistake of letting me knowI was now welcome to bang on his door every time I enter had scared the crap out of him. Slowly, more staff have put themselves in my crosshairs by thinking it'd be funny to get me first decided to join in the fun. I have one rule: Administrators are off limits...except Mrs. H. But, she started it. She got me good.
Problem is, I can't scare the woman. She has this mother-grandma-teacher-administrator-eyes-in-the-back-of-my-head-I-feel-a-disturbance-in-the-force kinda Jedi thing going. I can't sneak up on her. This morning, I spotted her about 100' away. Her back was turned. Kids were walking around everywhere. Conditions were perfect. There was no chance she saw me. None. I get 10' away and she turns and says, "Good morning, David."
"Dangit! How do you do that?" (I think I actually jumped up and down while I said that.)
I'm not giving up. I'll get her someday. But in the meantime, she better keep a close eye on her stapler.
Like, maybe, accidentally leaving the guidance office with things that happen to belong on the secretaries' desks only to return them in the form of gifts on Secretary Appreciation Day in the Spring. We all get a good laugh out of it--except that one time a fight almost broke out because Mrs. P thought Ms. A had taken her tape dispenser.
Or, rearranging the furniture in a teacher's room while they're out on school business. (Note: don't do that one again.)
Or dressing up like a colleague on Nerd Day. (Well, I thought it was funny.)
Most of these antics were the result of me being part of a cohort of younger teachers who were now teaching at the same high school we had attended.
Things changed when I moved to the new middle school. Most of the "new" staff had come from the same school, so I had to take some time adjusting to the culture that many had brought with them. Seemed like the right thing to do. As a result, I quickly became good friends with Mr. G, who had come to us by way of a neighboring district. We'd usually end up in one of our rooms by the end of the lunch period. But one day, I walked into his room and he had a visitor. I felt like I had kind of intruded so the next time I walked up there, I knocked as I entered to let him know I was coming. He made the mistake of letting me know
Problem is, I can't scare the woman. She has this mother-grandma-teacher-administrator-eyes-in-the-back-of-my-head-I-feel-a-disturbance-in-the-force kinda Jedi thing going. I can't sneak up on her. This morning, I spotted her about 100' away. Her back was turned. Kids were walking around everywhere. Conditions were perfect. There was no chance she saw me. None. I get 10' away and she turns and says, "Good morning, David."
"Dangit! How do you do that?" (I think I actually jumped up and down while I said that.)
I'm not giving up. I'll get her someday. But in the meantime, she better keep a close eye on her stapler.
Wednesday, September 15, 2010
If Only It Was Always Like This
Instead of working through the problems in the geometry book, we decided it would be fun to try to prove all the theorems as we come to them. A couple of weeks ago he proved the midsegment theorem. Now we are on to trying to prove that the centroid is 2/3 of the way down the median. So we sit around the white board easel and discuss how we might go about this. At this point, I don't even know how we're gonna prove this thing. We learned from the midsegment theorem that defining the vertices using (x1, y1), (x2, y2), (x3, y3) helped out greatly. So, what the heck, let's try it again.
We know we can define the midpoints generally as well, so that's what we do. Then it hits! We can define the vector from the vertex to the midtpoint of the opposite side, use a scalar of 2/3 to determine the vector from the vertex to what's supposed to be the centroid and then translate the vertex to said point.
Ta-freakin'-da
Turns out that the centroid is 1/3(x1+ x2+x3, y1+ y2+y3).
I didn't know that. If this is what it's like to become a co-learner with your students, then sign me the heck up.
We know we can define the midpoints generally as well, so that's what we do. Then it hits! We can define the vector from the vertex to the midtpoint of the opposite side, use a scalar of 2/3 to determine the vector from the vertex to what's supposed to be the centroid and then translate the vertex to said point.
Ta-freakin'-da
Turns out that the centroid is 1/3(x1+ x2+x3, y1+ y2+y3).
I didn't know that. If this is what it's like to become a co-learner with your students, then sign me the heck up.
Tuesday, September 14, 2010
WCYDWT: Space Shuttle Discovery
I've tipped my hand here a bit because simply showing a shuttle launch would be way too open ended. I have the original lesson plan as designed by NASA and I'm pretty sure how I'd go about presenting this lesson, but I'm curious as to how y'all'd do it.
STS 121 Launch (Initial/Final values) from David Cox on Vimeo.
STS 121 Launch (Initial/Final values) from David Cox on Vimeo.
Thursday, September 2, 2010
How Much is TOO Much?
What else can I ask him to do?
He's 13.
I mean, c'mon. I can ask for a more rigorous proof, but the kid can manipulate these expressions and then say things like, "I think this looks really cool with all x's and y's all over the place. "
He's 13.
I mean, c'mon. I can ask for a more rigorous proof, but the kid can manipulate these expressions and then say things like, "I think this looks really cool with all x's and y's all over the place. "
Thursday, August 26, 2010
Exponent Rules
I've grown tired of kids blindly following rules. Mine have the tendency to be the worst because they have always been really good at playing school. So we went for a different approach to exponent rules this week.
Prerequisites: Basic understanding of exponents
Instructions: Choose a rule that you would like to prove (read: demonstrate why it is a rule). As you are able to demonstrate an exponent rule, move downstream to the next group and help with that rule finally working on rule #8.
Here's the list...
...that culminates with the question:
[1]This conversation took about 12 minutes with the camera being shut off a couple of times. I limited the editing so as to try to capture the classroom vibe as naturally as possible. I asked a few questions that I'd like to take back, but... you live and learn.
Prerequisites: Basic understanding of exponents
Instructions: Choose a rule that you would like to prove (read: demonstrate why it is a rule). As you are able to demonstrate an exponent rule, move downstream to the next group and help with that rule finally working on rule #8.
Here's the list...
8. What do you think x1/2 represents?
I'll let them tell you.[1]
[1]This conversation took about 12 minutes with the camera being shut off a couple of times. I limited the editing so as to try to capture the classroom vibe as naturally as possible. I asked a few questions that I'd like to take back, but... you live and learn.
Wednesday, August 25, 2010
Proper-tays
I don't usually enjoy teaching properties because they seem so math-y. I like asking my kids to justify what they do, but for many, the properties just seem to be vocabulary that is forced upon them. Necessary evil, I guess? They are great for doing mental math tricks and kids use them without thinking of them, so I suppose there is no harm in giving a name to the stuff they already do.
Raise your hand if your kids mix up associative and commutative properties?
No more.
The Process
1. Give example of property with respect to addition.
ex: Associative: (2 + 3) + 4 = 2 + (3 + 4)
2. Ask students to write another example of their own.
3. Ask for a rule using a, b and c.
4. Ask students to write down the key characteristics of the associative property in Tweet form. (very few words)
Now here's the kicker:
5. Can you guess what the property for multiplication is going to look like?
This worked great for the associative, commutative and identity properties. A great discussion on the inverse property ensued and I ended up telling them that we want a multiplication problem that equals 1.
Done.
None of these properties are worth anything if we don't apply them. Next step is getting them to put words to all that stuff they "just do in my head."
6.
Let's synthesize this a bit more.
7. Now write a similar problem using the multiplicative properties.
I told the class to keep an eye out for times when we will use the inverse and identity properties--which will happen daily once we start solving equations.
Raise your hand if your kids mix up associative and commutative properties?
No more.
The Process
1. Give example of property with respect to addition.
ex: Associative: (2 + 3) + 4 = 2 + (3 + 4)
2. Ask students to write another example of their own.
3. Ask for a rule using a, b and c.
4. Ask students to write down the key characteristics of the associative property in Tweet form. (very few words)
Now here's the kicker:
5. Can you guess what the property for multiplication is going to look like?
This worked great for the associative, commutative and identity properties. A great discussion on the inverse property ensued and I ended up telling them that we want a multiplication problem that equals 1.
Done.
None of these properties are worth anything if we don't apply them. Next step is getting them to put words to all that stuff they "just do in my head."
6.
Let's synthesize this a bit more.
7. Now write a similar problem using the multiplicative properties.
I told the class to keep an eye out for times when we will use the inverse and identity properties--which will happen daily once we start solving equations.
Saturday, August 21, 2010
Toy Cars
Prompt: How far would you have to pull the car back in order to get it to go 100'?
Materials: Toy cars, meter sticks.
Hand them the cars, ask the question and get out of the way.
Question #1
But Mr. Cox, the farthest we can get the car to go is around 10'. We can only pull it back so far until it starts clicking.
Right. So if you could build a car that could be pulled back farther, how far would you need in order for the car to go 100'?
Question #2
Mr Cox, what do we do if our car keeps turning?
Yeah, I'm
The two groups that had problems with the car came up with two different solutions. One group decided to tie a piece of string to the spoiler and measured the amount of string the car pulled past the starting line and the other group simply estimated by breaking the curve down into short line segments. (Oh man, do you guys just realized you set me up for a lesson plan in May? Can you say calculus?)
Question #3
Mr. Cox, if I pull the car back 3", it goes 30", but if she pulls it back 3", it goes 36". Why?
Turns out that one kid pushed down on the car harder than the other which kept the tires from sliding.
Our Findings
I'm not sure if it is supposed to be or not, but the data was pretty linear. One student wondered why it would be linear since the car takes time to speed up and slow down and the shorter distance it travels, the more energy it is using to simply get up to speed.
Reason #421 Twitter is awesome
I tweet some pics of the activity and Frank asks me if I'm going to have a contest to see which group can get their car closest to the line.
*ahem* Of course I'm going to have a contest at the end to see who can best predict the distance their car travels.
Three groups were able to get within 1.5". (Two of them were the groups whose cars turned). The best was this:
Tuesday, August 3, 2010
Mixed Meta Four
If you haven't read about the Wolverine[1] (here and here) or Sam's Exasperating Problem, you need to get your priorities straight go read 'em now. Sam's wondering if the problem can be scaled down so a precalculus class can handle it. I see the problem and think, "GEOGEBRA! I CAN USE GEOGEBRA!" Mr. H beat me to it. (If you haven't seen his applet, I question your dedication to the cause go ahead, we'll wait.)
I love this! We are always looking for ways to iterate problems and extend them, but there's nothing to extend with this problem. It's all ready for the wolverine wrangler to do his stuff. I'm looking for the guy who can make this wolverine sit and quit bearing its teeth so my 8th graders can pet it for a second. GeoGebra does this. Mr. H's applet makes this problem accessible to an 8 year old. In fact, my son was so mesmerized by the animation that I swear I heard him muttering, "Heffalumps and Woozles. Heffalumps and Woozles." Heck, I found a strange urge to put on some Pink Floyd myself.
Can you imagine starting a problem in middle school and finishing it with calculus? That's how beautiful (that's right I said it!) this problem is. Why can't we let these younger kids see the beauty of the wolverine without actually having to be the one to handle it? I can see posing the problem, setting the kids up with GeoGebra (with minimal prerequisites) and turning them loose. They'll see the pattern, make a conjecture and inductively decide the answer. Show the applet which demonstrates the first 360 cases and inevitably, the question will be:
Now, talk about storytelling. The table's been set for the sequel that the kid's gonna have to wait a couple of years to see. precalculus kids can actually calculate the answer and the trilogy will be complete once they have the tools to actually prove that for n chords, the product is n+1. This problem can span four years. At least.
[1] Apologies if I misused the metaphor.
I love this! We are always looking for ways to iterate problems and extend them, but there's nothing to extend with this problem. It's all ready for the wolverine wrangler to do his stuff. I'm looking for the guy who can make this wolverine sit and quit bearing its teeth so my 8th graders can pet it for a second. GeoGebra does this. Mr. H's applet makes this problem accessible to an 8 year old. In fact, my son was so mesmerized by the animation that I swear I heard him muttering, "Heffalumps and Woozles. Heffalumps and Woozles." Heck, I found a strange urge to put on some Pink Floyd myself.
Can you imagine starting a problem in middle school and finishing it with calculus? That's how beautiful (that's right I said it!) this problem is. Why can't we let these younger kids see the beauty of the wolverine without actually having to be the one to handle it? I can see posing the problem, setting the kids up with GeoGebra (with minimal prerequisites) and turning them loose. They'll see the pattern, make a conjecture and inductively decide the answer. Show the applet which demonstrates the first 360 cases and inevitably, the question will be:
Why?!
Now, talk about storytelling. The table's been set for the sequel that the kid's gonna have to wait a couple of years to see. precalculus kids can actually calculate the answer and the trilogy will be complete once they have the tools to actually prove that for n chords, the product is n+1. This problem can span four years. At least.
[1] Apologies if I misused the metaphor.
Tuesday, July 27, 2010
Creating a Culture of Questions
Virtual Conference on Soft Skills [1]
[Note: This post was pretty much written by all of you. There are no unique ideas here (save for a few anecdotes) but I still found it productive to try to flesh out exactly what it is that I do to promote this culture of questions in my classroom. It's a huge part of what I do, but I'm afraid my ability to articulate the process may be lacking. I asked for a bunch of feedback via facebook/email from former students for this; kids ranging from the classes of '99 to '14 and they really helped shape this post.] So here it goes:
I learned how to learn when I was in college. No one told me. It just happened. As a teacher I have tried to help this process along a bit for my students because it kinda pissed me off that I spent 14 years in school and no one actually told me, "Learning is about the questions you ask, not the answers." So that pretty sums up my teaching philosophy. It hasn't changed much in 16 years.
Kids don't get that. They think that as long as they get the right answer, who cares about the how and the why? Questions and answers are on opposite teams. Answers get my work put on the refrigerator. Questions mean I don't know the answers. Answers mean I know. Questions mean I don't know. I can't let 'em know I don't know.
That's wrong. It's our job to change that. It's not easy and you have to set the table for yourself. The pillars to a questioning classroom involve: Truth, Trust, Togetherness and Transparency.
Truth
I talk to my students. A lot. In just about every year I've taught, I've had some variation of the same discussion with my classes. For some classes, this talk happens in the first week. For others it doesn't happen until the second semester. But inevitably, it happens.
It goes something like this:
Suppose all the information in the world is contained in this circle.
Here's you.
Some of them get offended until I show them this:
We're kinda in the same boat. Not much to brag about and not much to be embarrassed about.
And the more we learn, the sooner we realize that this circle is waaaay too small.
If our goal is to learn everything there is to learn, then we are chasing a finish line that's moving away from us much faster than we're moving towards it.
The only way we begin to know is when we realize that we don't know...and become OK with that.
So, how do we share our knowledge?
Questions.
Trust
Once we've established that we're all really in the same boat, there's no excuse for false pride. It's time to build trust. Do I really believe what I told them? I make it very clear to my students that I'm not smarter than they are; I just have more experience. I make it very clear that I don't tolerate anyone looking down on others for asking questions. I'm not so much of a there's no such thing as a dumb question kinda guy as much as I am a who are you to look down on someone who doesn't know? You didn't know the first time you tried kinda guy. This always leads to a digression about the first time we all tried to walk or ride a bike (my kids turn out to be great fodder for these kinds of discussions) which furthers the trust factor as I let them into my life a bit.
I don't let my students say, "That's easy" when someone asks a question because it deflates the questioner. Quickly. First time that comes out of someone's mouth I say, "Everything's easy once you get it."
I have to be honest, establishing trust was much easier for me when I taught high school. It's been a bit more of a challenge with 7th and 8th graders because I have a Kate-ish thing for truth. Sometimes I have to dial it back a bit without becoming falsely warm and fuzzy.
Togetherness
It takes some students quite a while to adapt to my questioning style in class. I've had kids want to drop my class (especially when I was at the high school) because"he doesn't give me the answers" "he never answers my questions." It's tough sometimes because kids are resolute. They'll try to corner you into taking the pencil out of their hand. The key is consistency. The more questions I ask, the more willing they are to ask.
One of the things I've done to help establish a willingness to ask questions is give the class lateral thinking puzzles. I mean, c'mon, there's no way you can guess the answer on some of these on the first try. Asking questions and being wrong are part of the process because they help us eliminate potential possibilities. It takes kids time to figure this out because they want to be specific with their questions at first which is very counterproductive. They have to learn to start with very general questions and the yes/no answer tells them whether or not to continue down that path. Once they get the hang of this, they start to realize that there are common themes in many of these puzzles which can be applied to later puzzles. This works nicely with problem solving strategies down the road.
At some point I throw out the challenge for them to find a lateral thinking puzzle that will stump me, which can't be done (or so I say). The trash talk ensues and I model the heck out of how one goes from the very general question to more specific questions.
The important thing here is that we do these as a class, together.
Transparency
Transparency has two meanings here: The I'm-not-here-to-trick-you-here's-really-what-I expect kind of transparency and the physical-posture-I'll-take-in-class-so-become-invisible kind of transparency.
Expectations
Expectations/standards/topics...whatever, have to be absolutely clear. The What? can't change. It's the How? that is up for discussion. I try things. I show my students first hand that I don't have all the answers and I am constantly trying to find better ways to help them learn. I fail. A lot. That's because I don't believe I have to have all the kinks worked out before I do something with my students. Our entire class is one big question and that question is this:
What do we need to do in order for us to learn?
Invisibility
I don't have just one (physical) focal point in my room. I have a SmartBoard on one wall, a dry erase board on the adjacent wall, multiple dry erase easels spread out throughout the room and I roam around using a wireless tablet that lets me annotate my slides. The focal point is the content, not a person. Students need to learn that the teacher is just one of many resources and doesn't necessarily have to be the primary resource. This means that I have to make myself invisible at times. I usually walk to the opposite side of the room as the person who is talking or maybe I actually take a seat during discussion and stare at the floor. I've found that kids start to depend on each other if I'm not there (so to speak). And when they depend on each other, they tend to start asking questions.
So, I guess at the end of the day, I try to be as real with my students as I can. This all comes down to relationships founded on truth; a truth that we can only catch glimpses of. We often times beat ourselves up because we don't see the fruit of our labor. These "soft skills" (who coined that term, anyway?) are really the reason we do what we do. We spend a copious number hours finding ways to offer immediate feedback to our students but our feedback is much more slow cookin'. We won't know if the time we spend with our kids will pay them dividends down the road, especially when it comes to these "soft skills." That comes when we see our students after they have finished college (or maybe they didn't go to college and went straight to work) and started their own families. That's when we see the fruit. So be patient, the harvest is comin'.
[1] Huge thanks to Riley for putting this together. The posts that have been assembled have been phenomenal and everyone who took the time to put something together should be commended.
Update (as per Dave's request)
My class layout. I teach in a an "art room" but since we don't offer classes like that anymore, I'm in it. Students sit in pods (equipped with outlets making computer time nice), 4 or 5 to a group depending on class size. I'm hardly in the same place for more than 5 minutes.
[Note: This post was pretty much written by all of you. There are no unique ideas here (save for a few anecdotes) but I still found it productive to try to flesh out exactly what it is that I do to promote this culture of questions in my classroom. It's a huge part of what I do, but I'm afraid my ability to articulate the process may be lacking. I asked for a bunch of feedback via facebook/email from former students for this; kids ranging from the classes of '99 to '14 and they really helped shape this post.] So here it goes:
I learned how to learn when I was in college. No one told me. It just happened. As a teacher I have tried to help this process along a bit for my students because it kinda pissed me off that I spent 14 years in school and no one actually told me, "Learning is about the questions you ask, not the answers." So that pretty sums up my teaching philosophy. It hasn't changed much in 16 years.
Kids don't get that. They think that as long as they get the right answer, who cares about the how and the why? Questions and answers are on opposite teams. Answers get my work put on the refrigerator. Questions mean I don't know the answers. Answers mean I know. Questions mean I don't know. I can't let 'em know I don't know.
That's wrong. It's our job to change that. It's not easy and you have to set the table for yourself. The pillars to a questioning classroom involve: Truth, Trust, Togetherness and Transparency.
Truth
I talk to my students. A lot. In just about every year I've taught, I've had some variation of the same discussion with my classes. For some classes, this talk happens in the first week. For others it doesn't happen until the second semester. But inevitably, it happens.
It goes something like this:
Suppose all the information in the world is contained in this circle.
Here's you.
Some of them get offended until I show them this:
What happens when we share?
And the more we learn, the sooner we realize that this circle is waaaay too small.
If our goal is to learn everything there is to learn, then we are chasing a finish line that's moving away from us much faster than we're moving towards it.
The only way we begin to know is when we realize that we don't know...and become OK with that.
So, how do we share our knowledge?
Questions.
Trust
Once we've established that we're all really in the same boat, there's no excuse for false pride. It's time to build trust. Do I really believe what I told them? I make it very clear to my students that I'm not smarter than they are; I just have more experience. I make it very clear that I don't tolerate anyone looking down on others for asking questions. I'm not so much of a there's no such thing as a dumb question kinda guy as much as I am a who are you to look down on someone who doesn't know? You didn't know the first time you tried kinda guy. This always leads to a digression about the first time we all tried to walk or ride a bike (my kids turn out to be great fodder for these kinds of discussions) which furthers the trust factor as I let them into my life a bit.
I don't let my students say, "That's easy" when someone asks a question because it deflates the questioner. Quickly. First time that comes out of someone's mouth I say, "Everything's easy once you get it."
I have to be honest, establishing trust was much easier for me when I taught high school. It's been a bit more of a challenge with 7th and 8th graders because I have a Kate-ish thing for truth. Sometimes I have to dial it back a bit without becoming falsely warm and fuzzy.
Togetherness
It takes some students quite a while to adapt to my questioning style in class. I've had kids want to drop my class (especially when I was at the high school) because
One of the things I've done to help establish a willingness to ask questions is give the class lateral thinking puzzles. I mean, c'mon, there's no way you can guess the answer on some of these on the first try. Asking questions and being wrong are part of the process because they help us eliminate potential possibilities. It takes kids time to figure this out because they want to be specific with their questions at first which is very counterproductive. They have to learn to start with very general questions and the yes/no answer tells them whether or not to continue down that path. Once they get the hang of this, they start to realize that there are common themes in many of these puzzles which can be applied to later puzzles. This works nicely with problem solving strategies down the road.
At some point I throw out the challenge for them to find a lateral thinking puzzle that will stump me, which can't be done (or so I say). The trash talk ensues and I model the heck out of how one goes from the very general question to more specific questions.
The important thing here is that we do these as a class, together.
Transparency
Transparency has two meanings here: The I'm-not-here-to-trick-you-here's-really-what-I expect kind of transparency and the physical-posture-I'll-take-in-class-so-become-invisible kind of transparency.
Expectations
Expectations/standards/topics...whatever, have to be absolutely clear. The What? can't change. It's the How? that is up for discussion. I try things. I show my students first hand that I don't have all the answers and I am constantly trying to find better ways to help them learn. I fail. A lot. That's because I don't believe I have to have all the kinks worked out before I do something with my students. Our entire class is one big question and that question is this:
What do we need to do in order for us to learn?
Invisibility
I don't have just one (physical) focal point in my room. I have a SmartBoard on one wall, a dry erase board on the adjacent wall, multiple dry erase easels spread out throughout the room and I roam around using a wireless tablet that lets me annotate my slides. The focal point is the content, not a person. Students need to learn that the teacher is just one of many resources and doesn't necessarily have to be the primary resource. This means that I have to make myself invisible at times. I usually walk to the opposite side of the room as the person who is talking or maybe I actually take a seat during discussion and stare at the floor. I've found that kids start to depend on each other if I'm not there (so to speak). And when they depend on each other, they tend to start asking questions.
So, I guess at the end of the day, I try to be as real with my students as I can. This all comes down to relationships founded on truth; a truth that we can only catch glimpses of. We often times beat ourselves up because we don't see the fruit of our labor. These "soft skills" (who coined that term, anyway?) are really the reason we do what we do. We spend a copious number hours finding ways to offer immediate feedback to our students but our feedback is much more slow cookin'. We won't know if the time we spend with our kids will pay them dividends down the road, especially when it comes to these "soft skills." That comes when we see our students after they have finished college (or maybe they didn't go to college and went straight to work) and started their own families. That's when we see the fruit. So be patient, the harvest is comin'.
[1] Huge thanks to Riley for putting this together. The posts that have been assembled have been phenomenal and everyone who took the time to put something together should be commended.
Update (as per Dave's request)
My class layout. I teach in a an "art room" but since we don't offer classes like that anymore, I'm in it. Students sit in pods (equipped with outlets making computer time nice), 4 or 5 to a group depending on class size. I'm hardly in the same place for more than 5 minutes.









3.jpg)
.jpg)
2.jpg)
3.jpg)
.jpg)



.jpg)