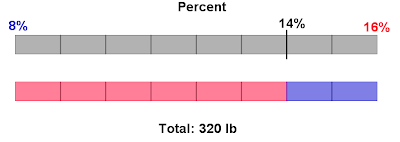
Soybean meal is 16% protein and cornmeal is 8% protein. How many lbs. of each should be added to get a 320 lb mix that is 14% protein?
I've never been a big fan of 8th grade students having to work through mixture problems, but maybe that has to do with the way I've taught it.
Every year I would come up with a new way to encourage students to set up equations to solve these problems but we'd always end up with some variation of this:
Let x = lb of cornmeal
Let y = lb of soybean meal
x + y = 320
.08x + .16y = .14(320)
Solve for x and y.
And for my advanced classes, that was fine. They already knew how to solve systems of equations and it just became another jumpable (yeah, that's a new word. Deal with it.) hoop.
It never set well with me.
This year, it kinda pisses me off. I've got kids who are able to think, but this kind of abstraction just kills them. It may seem intuitive to freaks like us, but for most kids, it's just a ridiculous application of an already ridiculous skill.
Enter proportions.
We live in the agricultural capital of the world. People mix stuff here all the time--and they don't use systems of equations to do it. They use common-freakin'-sense.
Mira.
Step 1
Step 2
Step 3
Step 4
Step 5
Sit back and relax while the other
I find this not only easier for students to do, but it appeals to a skill (proportional reasoning) that they may actually use 10 years from now as opposed to a skill (systems of equations) that they will use for about however long it takes to pass the test.
Question
How do I introduce this so that it appeals to my students' intuition in a way that keeps it from being just another trick they learn?
Applet is available for download or online use here.