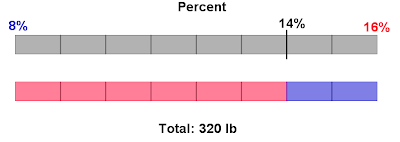
Soybean meal is 16% protein and cornmeal is 8% protein. How many lbs. of each should be added to get a 320 lb mix that is 14% protein?
I've never been a big fan of 8th grade students having to work through mixture problems, but maybe that has to do with the way I've taught it.
Every year I would come up with a new way to encourage students to set up equations to solve these problems but we'd always end up with some variation of this:
Let x = lb of cornmeal
Let y = lb of soybean meal
x + y = 320
.08x + .16y = .14(320)
Solve for x and y.
And for my advanced classes, that was fine. They already knew how to solve systems of equations and it just became another jumpable (yeah, that's a new word. Deal with it.) hoop.
It never set well with me.
This year, it kinda pisses me off. I've got kids who are able to think, but this kind of abstraction just kills them. It may seem intuitive to freaks like us, but for most kids, it's just a ridiculous application of an already ridiculous skill.
Enter proportions.
We live in the agricultural capital of the world. People mix stuff here all the time--and they don't use systems of equations to do it. They use common-freakin'-sense.
Mira.
Step 1
Step 2
Step 3
Step 4
Step 5
Sit back and relax while the other
I find this not only easier for students to do, but it appeals to a skill (proportional reasoning) that they may actually use 10 years from now as opposed to a skill (systems of equations) that they will use for about however long it takes to pass the test.
Question
How do I introduce this so that it appeals to my students' intuition in a way that keeps it from being just another trick they learn?
Applet is available for download or online use here.







11 comments:
Perhaps this way:
1) start by drawing a graph of protein content vs amount of soy (from 0 to 320 pounds).
2) Note that the 8% is constant and that the extra protein from soy rather than corn is 8%.
3) The amount of extra protein needed is 6% of 320. Show where that comes on the line. Argue similar triangles to say that to get 6/8th of the way you need 6/8ths of 320 pounds, or 240 lbs.
That reasoning shows the where the ratios come from, and allow them to do problems that aren't simple round numbers (like needing 13.1% protein).
I like the applette. Here's how a few of us algebra teacher in berkeley teach mixtures: http://www.teachingchannel.org/videos/algebra-i-mixture-problems?fd=0
Using proportions instead of systems of equations has been amazingly successful as a teaching strategy.
@gas
I'm sure your method would be helpful, but I'm not sure I understand your instructions.
@piecrust
Thanks for the link. What adjustments do you make to the seesaw method when both amounts are unknown?
I like this! Never seen this method before, but here's how I'd structure it:
I would start by asking the kids to predict what would happen if they mixed equal parts of 8% and 16% concentration. Can they figure out that the effect would neutralize to 12%? Have them prove it using base-10 cubes and the meaning of percents.
And then, have them investigate what happens as they mix in more parts of the higher concentration (16%) to one part of the lower concentration (8%). Each time they can play with representing % concentration using colored base-10 cubes and justifying their calculations in a table tallying different types of cubes.
Hopefully (through organized data in a table) they can discover when it will reach 14% is when the ratio reaches 4:1. Ask them why that is, and give them an absolute value hint ("How far away is 14% from each of the original concentrations?"), and then send them on another investigation with different percentage mixtures to investigate whether their proportional hunch is true with respect to distances on the number line.
If they start to see a pattern after two investigations, discuss as a class and THEN tie this into systems of equations to demonstrate a potential usefulness of systems algebra. I don't think the algebra should be omitted entirely, only because some kids are more comfortable with the abstract reasoning than others, and they should benefit still from the multiple-mode exposure?
Anyway, thanks for the idea! I'll definitely try it out with my 8th-graders in the spring. :)
I agree that systems shouldn't be abandoned. I just see some kids struggling with the abstraction and know that having them use systems would be like handing a drowning person a glass of water.
I like your suggestions, though. Thanks.
As a secondary math teacher by training, I'm still not sure if I fully understand mixture problems, but that's not the question you asked.
Instead, I'll take issue -- agriculture capital of the world? CA vs. IA -- are we talking quality or quantity? game on! :)
@David:
Lemme make sure I understand your question and then I'll try to draw a model (though I'll maybe have to show it on my blog since I can only respond with text here). By 'both amounts unknown' do you mean: "A chemist mixes a 10% saline solution with a 20% salines solution to make 500 milliliters of a 16% saline solution" How many milliliters of each solution does the chemist mix together?"
Or if 'amounts' refers to something other than the 2 volumes, give me an example and I'll chew on it.
Oh...instead of that new chemistry problem, is your original soybean problem what you mean when both amounts are unknown?
"How many milliliters of each solution does the chemist mix together?""
Yeah, that's exactly what I'm talking about.
ok, i tried to do it justice on my blog. see what you think. i'm really impressed (jealous) with your geogebra programming skills. really cool stuff!
Looking through your approach had me wondering why we need a system. How about this for another possible approach. Replace the original problem with the easier equivalent: one mixture is 0% another 8% and I want 320 lbs of 6%.
Post a Comment